The precise definition of the limit
What is the precise definition of the limit?
The precise definition of a limit is something we use as a proof for the existence of a limit.
Let’s start by stating that ???f(x)??? is a function on an open interval that contains ???x=a??? but that the function doesn’t necessarily exist at ???x=a???. We can say that
???\lim_{x \to a} f(x)=L???
if for every number ???\epsilon>0??? there is some number ???\delta>0??? such that
???\left|f(x)-L\right|<\epsilon??? whenever ???0<\left|x-a\right|<\delta???
Hi! I'm krista.
I create online courses to help you rock your math class. Read more.
How to understand the precise definition of the limit
What does all this mean? Since the open interval includes ???a??? but doesn’t necessarily exist at ???a???, we’ll have to look at how the function behaves at it approaches ???a???. ???L??? just represents the value of the limit.
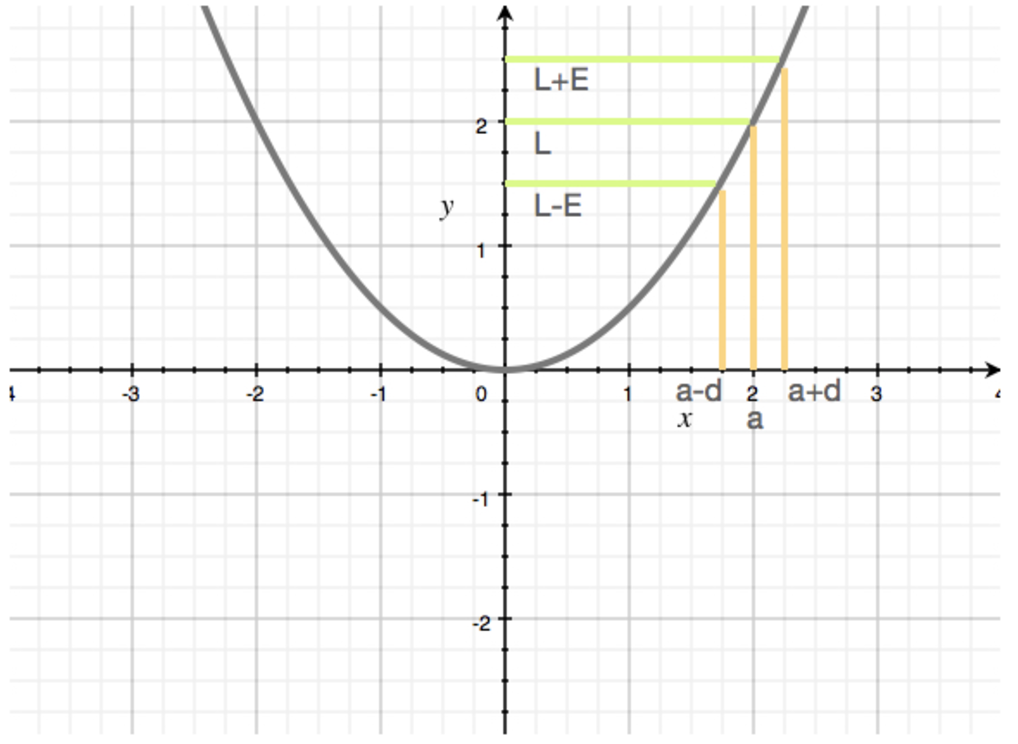
When we’re evaluating a limit, we’re looking at the function as it approaches a specific point. In the graph below, that point is ???(a,L)???. The precise definition of the limit proves that the limit exists and is ???L???, as long as any number we pick between ???a-\delta??? and ???a+\delta??? will always return a value between ???L-\epsilon??? and ???L+\epsilon???.
If this is true, then we know that, if we pick a value that’s closer and closer to ???a???, the value we get back will be closer and closer to ???L???. And that’s the definition of of the limit, right? That as we approach a particular value of ???x???, the function itself gets closer and closer to a particular value.
This is a video walkthrough of proving the value of the limit using the precise definition
Take the course
Want to learn more about Calculus 1? I have a step-by-step course for that. :)
How to prove the value of the limit with the precise definition
Example
Using the precise definition of the limit, prove the following limit.
???\lim_{x \to 4} 2x-3=5???
Substituting ???2x-3??? for ???f(x)???, ???5??? for ???L???, and ???4??? for ???a??? into the definition, we get
???\left|(2x-3)-5\right|<\epsilon??? whenever ???0<\left|x-4\right|<\delta???
The precise definition of a limit is something we use as a proof for the existence of a limit.
If we simplify ???\left|(2x-3)-5\right|<\epsilon???, we get
???\left|2x-8\right|<\epsilon???
???2\left|x-4\right|<\epsilon???
???\left|x-4\right|<\frac{\epsilon}{2}???
Notice now that the left side of this inequality looks just like the middle part of the inequality above that contains ???\delta???. When this happens we set ???\delta???equal to the right-hand side of the last inequality, and we get
???\delta=\frac{\epsilon}{2}???
???0<\left|x-4\right|<\delta=\frac{\epsilon}{2}???
Going back to the beginning,
???\left|(2x-3)-5\right|=\left|2x-8\right|???
???\left|(2x-3)-5\right|=2\left|x-4\right|???
and using the assumption that ???\delta=\frac{\epsilon}{2}??? and that ???0<\left|x-4\right|<\delta???, by substitution, we get
???\left|(2x-3)-5\right|=2\left|\frac{\epsilon}{2}\right|???
???\left|(2x-3)-5\right|=\epsilon???
Since we started with ???\left|(2x-3)-5\right|<\epsilon??? and ended with ???\epsilon??? we have shown that ???\epsilon=\epsilon??? and that
???\left|(2x-3)-5\right|<\epsilon??? whenever ???0<\left|x-4\right|<\frac{\epsilon}{2}???
Therefore,
???\lim_{x \to 4} 2x-3=5???