How to evaluate definite integrals with the Fundamental Theorem of Calculus

A definite integral asks how much a function accumulates over an interval. Once we find an antiderivative, we can answer that question by plugging in just two numbers. Let’s see where those numbers go and why we subtract.

Use the endpoint evaluation rule

If f is continuous on [a,b] and F is an antiderivative of f, evaluate F at the upper bound and subtract its value at the lower bound.

∫abf(x)dx=F(b)-F(a)

An antiderivative satisfies F′(x)=f(x). The definite integral gives a number. When the graph stays above the horizontal axis on the interval, that number is the area under the curve. Portions below the axis contribute negative signed area.

The two statements of the Fundamental Theorem are sometimes numbered in different orders. In the video, Part 1 refers to this fixed-bound evaluation rule.

Example: Evaluate the integral of a cubic

∫13x3dx

Start with the antiderivative F(x)=x44. Evaluate that same function at 3 and 1.

F(3)=344=814F(1)=144=14∫13x3dx=814-14=20
Area under y equals x cubed from one to threeThe cubic is above the horizontal axis on the interval. The region between the curve and the axis is shaded from x equals one to x equals three.13yxy = x³
The shaded region has area 20 square units.

Why the constant of integration cancels

We can choose any antiderivative, so we used the one with C=0. If we include the constant, the upper and lower values both contain the same C.

(814+C)-(14+C)=20

Before using endpoint evaluation, check the integrand over the whole interval. For example, 1x has a break at zero, so an integral across that point needs to be treated as an improper integral.

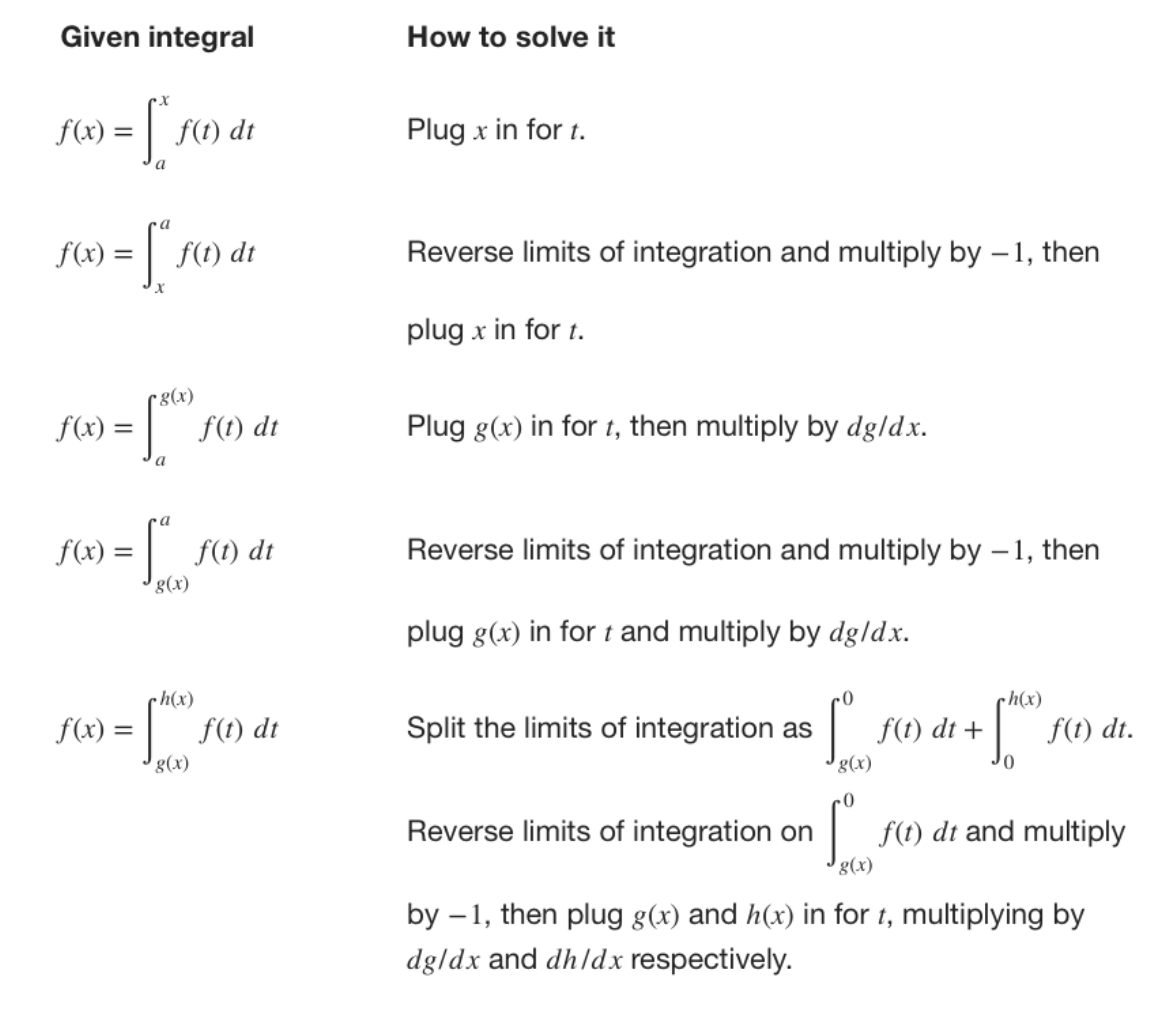
Learn mathKrista Kingmath online, fundamental theorem of calculus