One-sided limits
LIMITS & CONTINUITY
General vs. one-sided limits
When you hear your professor talking about limits, he or she is usually talking about the general limit. Unless a right- or left-hand limit is specifically specified, you’re dealing with a general limit.
The general limit exists at the point ???x=c??? if
1. The left-hand limit exists at ???x=c???,
2. The right-hand limit exists at ???x=c???, and
3. The left- and right-hand limits are equal.
When you hear your professor talking about limits, he or she is usually talking about the general limit.
These are the three conditions that must be met in order for the general limit to exist. The general limit will look something like this:
???\lim_{x\to 2}\ f(x)=4???
You would read this general limit formula as “The limit of ???f??? of ???x??? as ???x??? approaches ???2??? equals ???4???.”
Left- and right-hand limits may exist even when the general limit does not. If the graph approaches two separate values at the point ???x=c??? as you approach ???c??? from the left- and right-hand side of the graph, then separate left- and right-hand limits may exist.
Left-hand limits are written as
???\lim_{x\to 2^-}\ f(x)=4???
The negative sign after the ???2??? indicates that we’re talking about the limit as we approach ???2??? from the negative, or left-hand side of the graph.
Right-hand limits are written as
???\lim_{x\to 2^+}\ f(x)=4???
The positive sign after the ???2??? indicates that we’re talking about the limit as we approach ???2??? from the positive, or right-hand side of the graph.
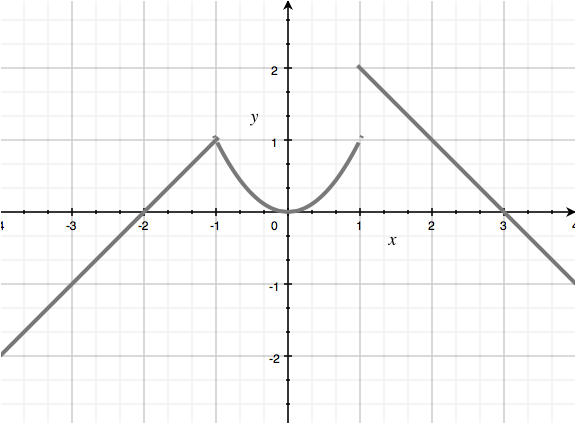
In the graph below, the general limit exists at ???x=-1??? because the left- and right- hand limits both approach ???1???. On the other hand, the general limit does not exist at ???x=1??? because the left-hand and right-hand limits are not equal, due to a break in the graph.
You can see from the graph that the left- and right-hand limits are equal at ???x=-1???, but not at ???x=1???.