Measures of parallelograms, including angles, sides, and diagonals
Defining all the measures of a parallelogram
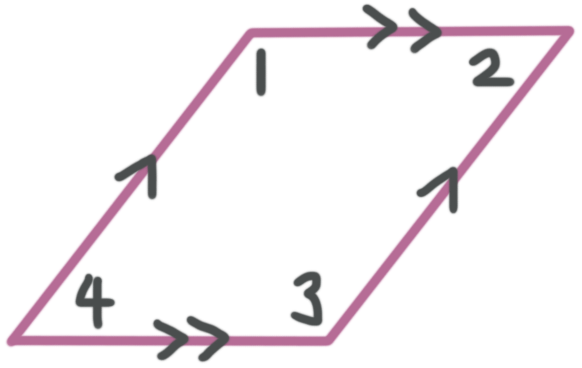
A parallelogram is a quadrilateral that has opposite sides that are parallel.
The parallel sides let you know a lot about a parallelogram.
Hi! I'm krista.
I create online courses to help you rock your math class. Read more.
Here are the special properties of parallelograms:
Parallelogram
Two pairs of opposite parallel sides
Opposite sides are equal lengths
Opposite angles are congruent
???m\angle 1=m\angle 3???
???m\angle 2=m\angle 4???
Consecutive angles are supplementary
???m\angle 1+m\angle 2=180^\circ???
???m\angle 2+m\angle 3=180^\circ???
???m\angle 3+m\angle 4=180^\circ???
???m\angle 4+m\angle 1=180^\circ???
Diagonals bisect each other (cut each other in half)
How to solve for every measure of a parallelogram, including angles, side lengths, and the lengths of diagonals
Take the course
Want to learn more about Geometry? I have a step-by-step course for that. :)
Finding the measure of a interior angle of a parallelogram
Example
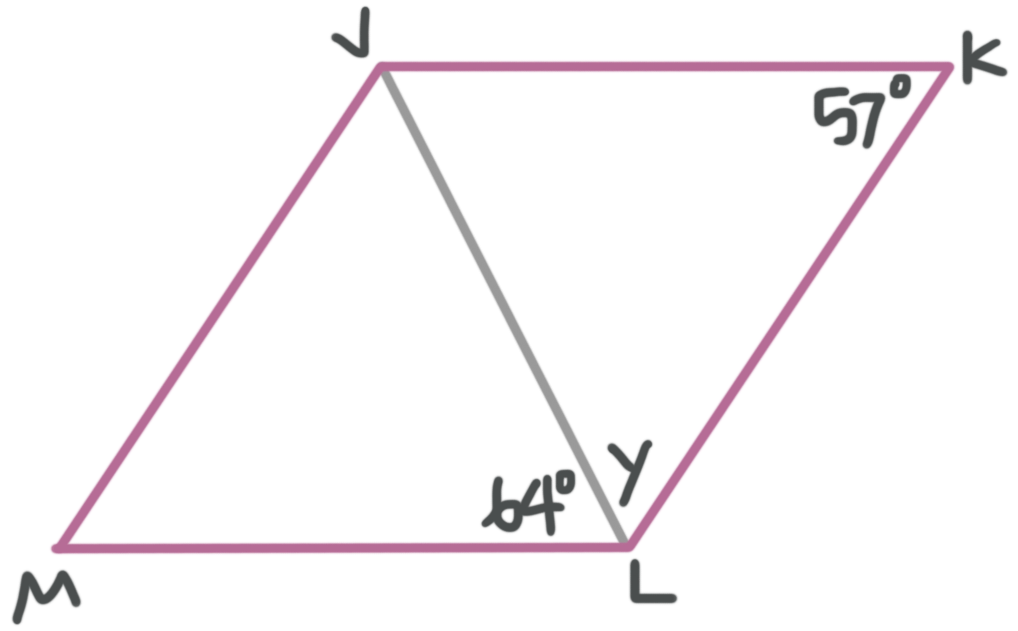
Find the measure of angle ???y???, given ???JKLM??? is a parallelogram.
Opposite angles of parallelograms are congruent, so
???m\angle JML=m\angle JKL=57^\circ???
Now we can use the fact that opposite sides of a parallelogram are parallel to state that ???JK\parallel ML???. This means that the diagonal ???JL??? of the parallelogram is also a transversal of these two parallel lines. This means that ???\angle KLJ??? and ???\angle MJL??? are alternate interior angles. Alternate interior angle pairs are congruent, so ???m\angle KLJ=m\angle MJL=y???.
The measures of the three interior angles of a triangle add up to ???180^\circ???, so we can set up an equation for the sum of the interior angles of ???\triangle JML??? and solve for ???y???.
???y+57^\circ+64^\circ=180^\circ???
???y=59^\circ???
A parallelogram is a quadrilateral that has opposite sides that are parallel.
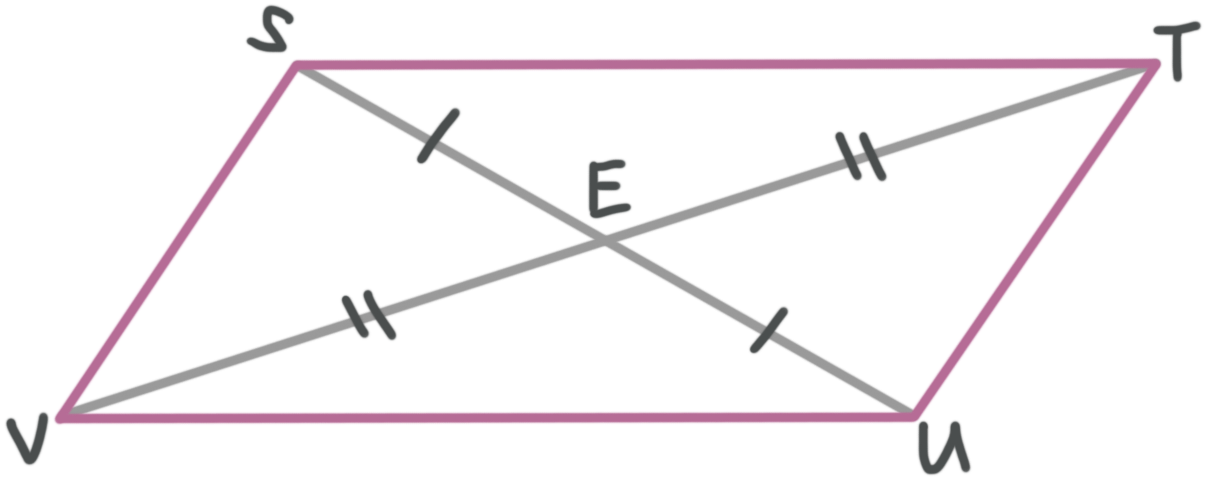
Example
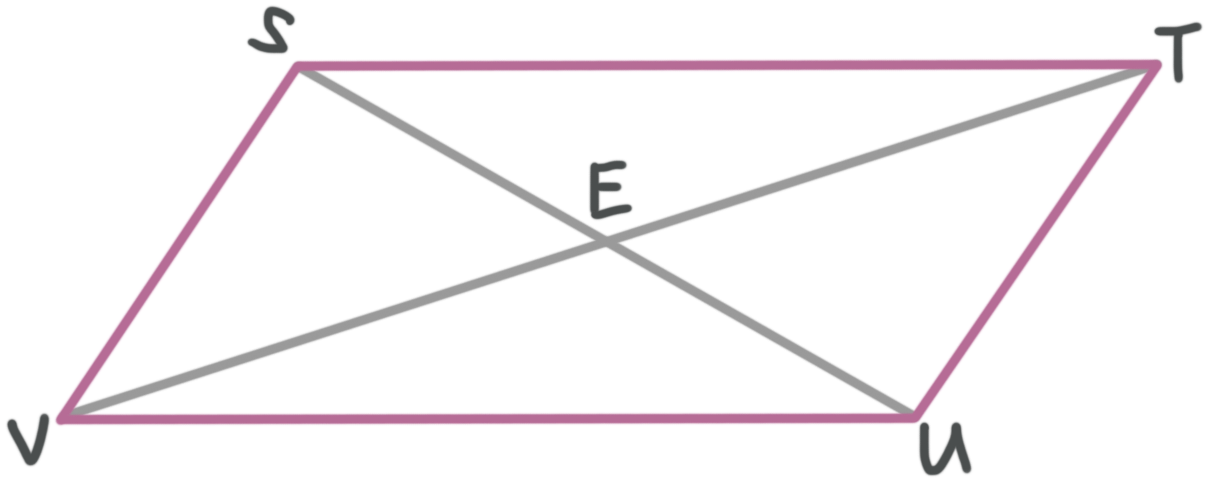
If ???STUV??? is a parallelogram, and if ???VT=4n+34??? and ???VE=7n-3???, what is the length of ???ET????
We know that the diagonals of a parallelogram bisect each other. Let’s add this information into the diagram.
Now we can see the relationships we need. Because the diagonals bisect, ???VE=ET??? and ???VE=(1/2)VT???. We can use what we know to find the length of ???VE??? and then we’ll know the length of ???ET??? as well.
???VE=\frac{1}{2}VT???
???7n-3=\frac{1}{2}(4n+34)???
???7n-3=2n+17???
???5n=20???
???n=4???
Now we can substitute back in to find the length of ???VE???, which is equal to the length of ???ET???.
???VE=ET=7n-3???
???VE=ET=7(4)-3???
???VE=ET=25???