Finding derivatives of hyperbolic functions
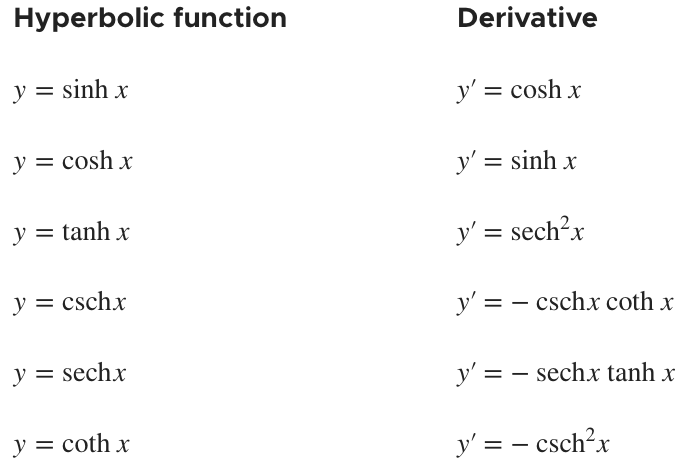
The derivatives of hyperbolic functions
We’ve looked at trig and inverse trig functions and their derivatives, and now we’ll look at hyperbolic and inverse hyperbolic trig functions and their derivatives in order to round out our lessons on derivatives with trig functions.
Fortunately, the derivatives of the hyperbolic functions are really similar to the derivatives of trig functions, so they’ll be pretty easy for us to remember. We only see a difference between the two when it comes to the derivative of cosine vs. the derivative of hyperbolic cosine.
Hi! I'm krista.
I create online courses to help you rock your math class. Read more.
The derivative of ???y=\cos{x}??? is ???y’=-\sin{x}???, but the derivative of ???y=\cosh{x}??? is ???y’=\sinh{x}???. Because the rest of the hyperbolic derivatives table follows the same pattern as the normal trig derivatives table, we’d expect the derivative of hyperbolic cosine to be ???y’=-\sinh{x}???, with the negative sign included. But its actual derivative doesn’t include the negative sign.
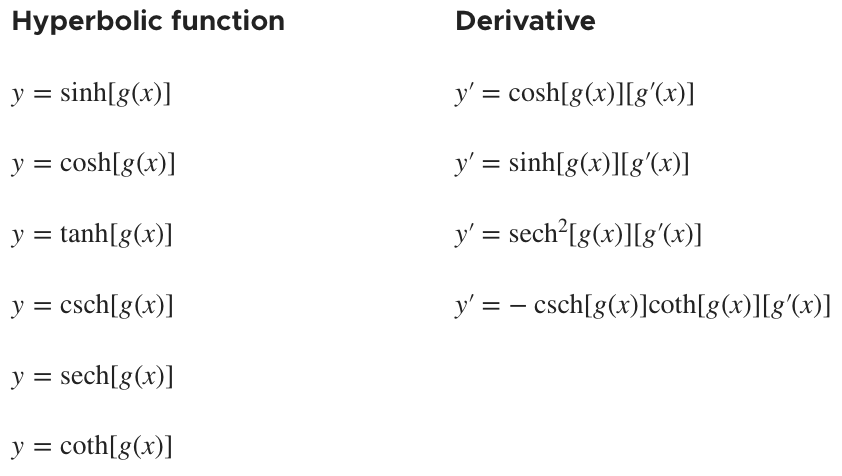
Just like with trig derivatives and inverse trig derivatives, we always apply chain rule and multiply by the derivative of the argument. In the table above, the argument is always ???x???, and since the derivative of ???x??? is ???1???. Of course, multiplying by ???1??? doesn’t affect the value of the derivative.
But if the argument is anything other than ???x???, then the derivative of the argument will be something other than ???1???, which means that applying the chain rule will affect the value of the derivative. When that’s the case, we can think of the hyperbolic derivative formulas as
Let’s work through a few examples so that we can get comfortable applying these derivative formulas.
How to calculate hyperbolic derivatives
Take the course
Want to learn more about Calculus 1? I have a step-by-step course for that. :)
Applying chain rule when we differentiate hyperbolic functions
Example
Find the derivative of the hyperbolic function.
???y=9\tanh{(x^5)}???
Apply the formula for the derivative of hyperbolic tangent.
???y'={\rm sech}^2{[g(x)]}[g'(x)]???
???y’=9{\text{sech}^2}{(x^5)}(5x^4)???
???y’=45x^4{\text{sech}^2}{(x^5)}???
Let’s try another example where the hyperbolic function occurs as part of a larger function.
Example
Find the function’s derivative.
???y=2\sinh{(4x^6)}-26x^2???
Take the derivative one term at a time, applying the formula for the derivative of hyperbolic sine to the first term,
???y'=\cosh{[g(x)]}[g'(x)]???
and power rule to the second term.
???y’=2\cosh{(4x^6)}(24x^5)-52x???
???y’=48x^5\cosh{(4x^6)}-52x???
Let’s try another more complex example.
Just like with trig derivatives and inverse trig derivatives, we always apply chain rule and multiply by the derivative of the argument.
Example
Find the derivative.
???y=8x^{-4}-{\text{csch}}{(3x^2)}+\frac{\sinh{(x^3)}}{\tanh{(2x^7)}}???
Let’s work on one term at a time. The derivative of the first term can be found using power rule.
???y' =8(-4)x^{-4-1}???
???y' =-32x^{-5}???
To find the derivative of the second term, we’ll apply the formula for the derivative of hyperbolic cosecant.
???y'=-{\rm csch}{[g(x)]}\coth{[g(x)]}[g'(x)]???
???y' = -{\rm csch}{(3x^2)}\coth{(3x^2)}(6x)???
???y' = -6x{\rm csch}{(3x^2)}\coth{(3x^2)}???
To find the derivative of the third term, we’ll use quotient rule, and apply the formulas for the derivatives of hyperbolic sine and hyperbolic tangent.
???y' = \frac {\cosh{(x^3)}(3x^2)\tanh{(2x^7)}-\sinh{(x^3)}{\rm sech}^2{(2x^7)}(14x^6)} {(\tanh{(2x^7)})^2}???
???y' = \frac {3x^2\cosh{(x^3)}\tanh{(2x^7)}-14x^6\sinh{(x^3)}{\rm sech}^2{(2x^7)}} {\tanh^2{(2x^7)}}???
Putting these derivatives together gives the derivative for the original function.
???y' = -32x^{-5} -6x{\rm csch}{(3x^2)}\coth{(3x^2)}???
???+\frac {3x^2\cosh{(x^3)}\tanh{(2x^7)}-14x^6\sinh{(x^3)}{\rm sech}^2{(2x^7)}} {\tanh^2{(2x^7)}}???